√70以上 find dy/dx if y=(x 1)(x 2) 161666-Find dy/dx if y=x^3-1/x+2

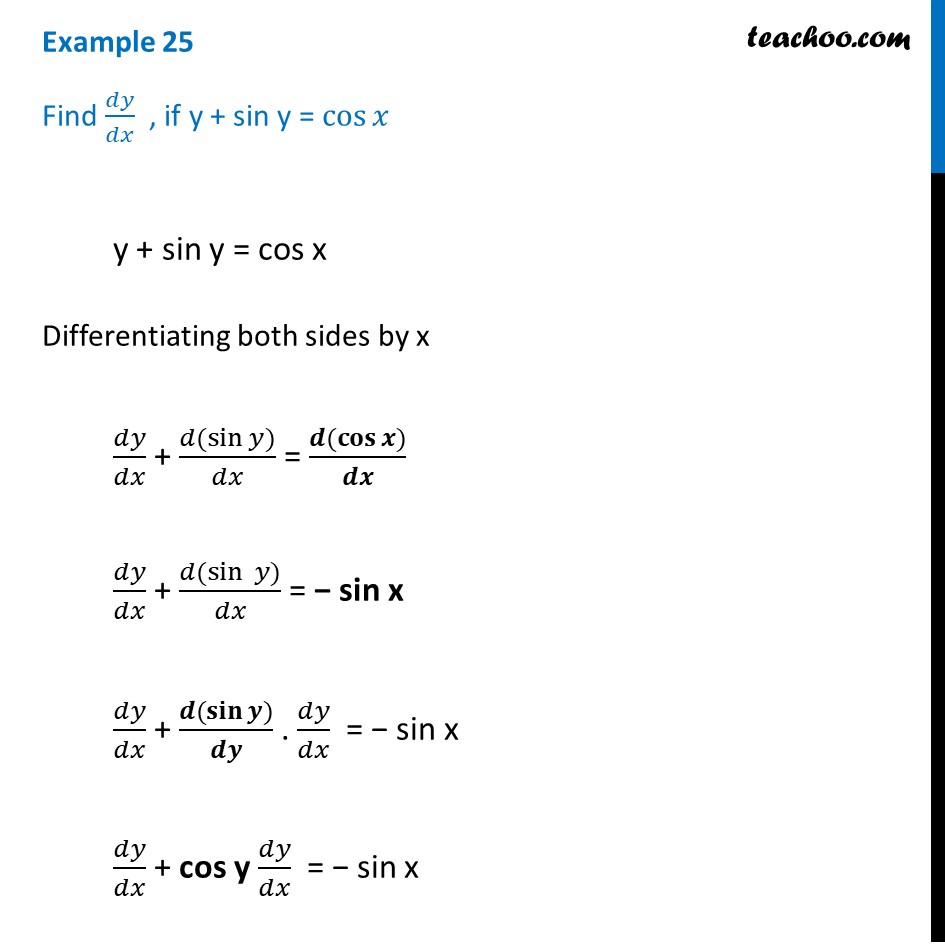
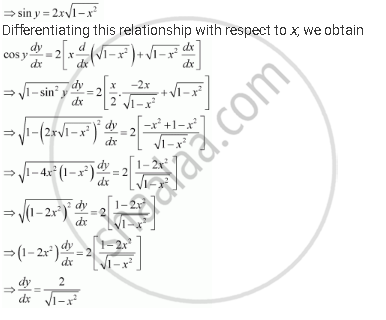
Inverse Functions Implicit differentiation can help us solve inverse functions The general pattern is Start with the inverse equation in explicit form Example y = sin −1 (x) Rewrite it in noninverse mode Example x = sin (y) Differentiate this function with respect to

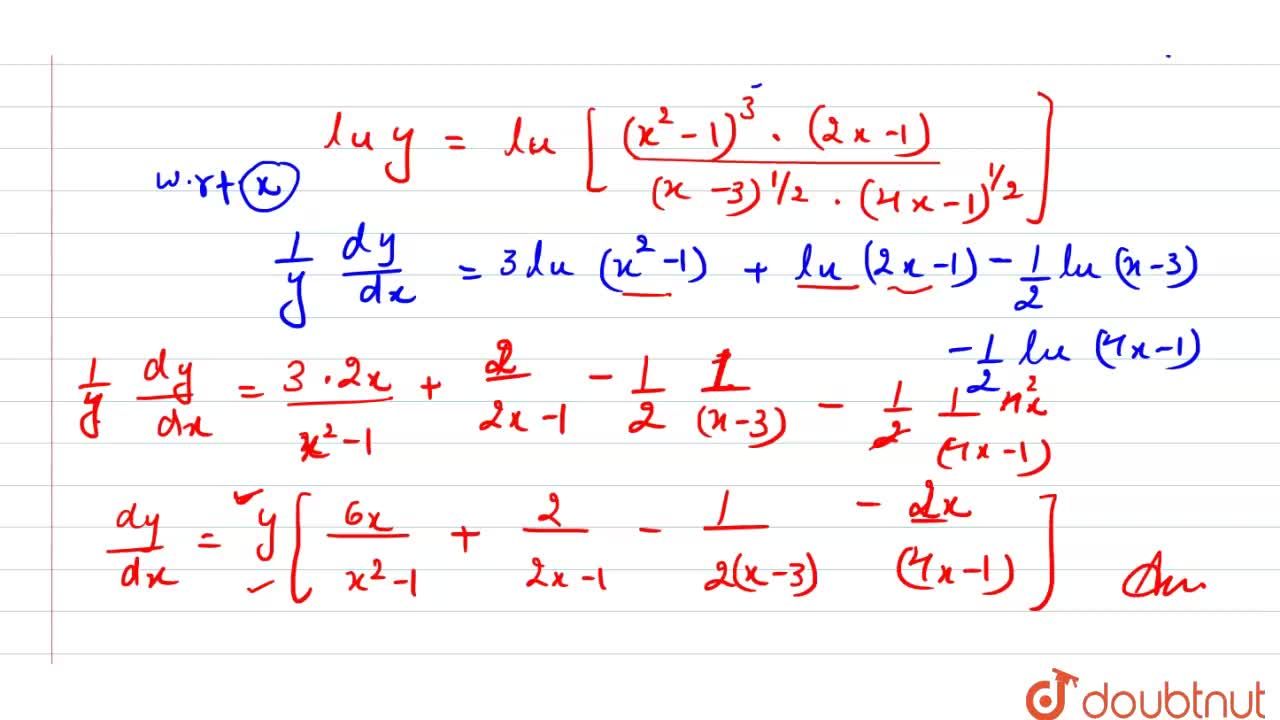
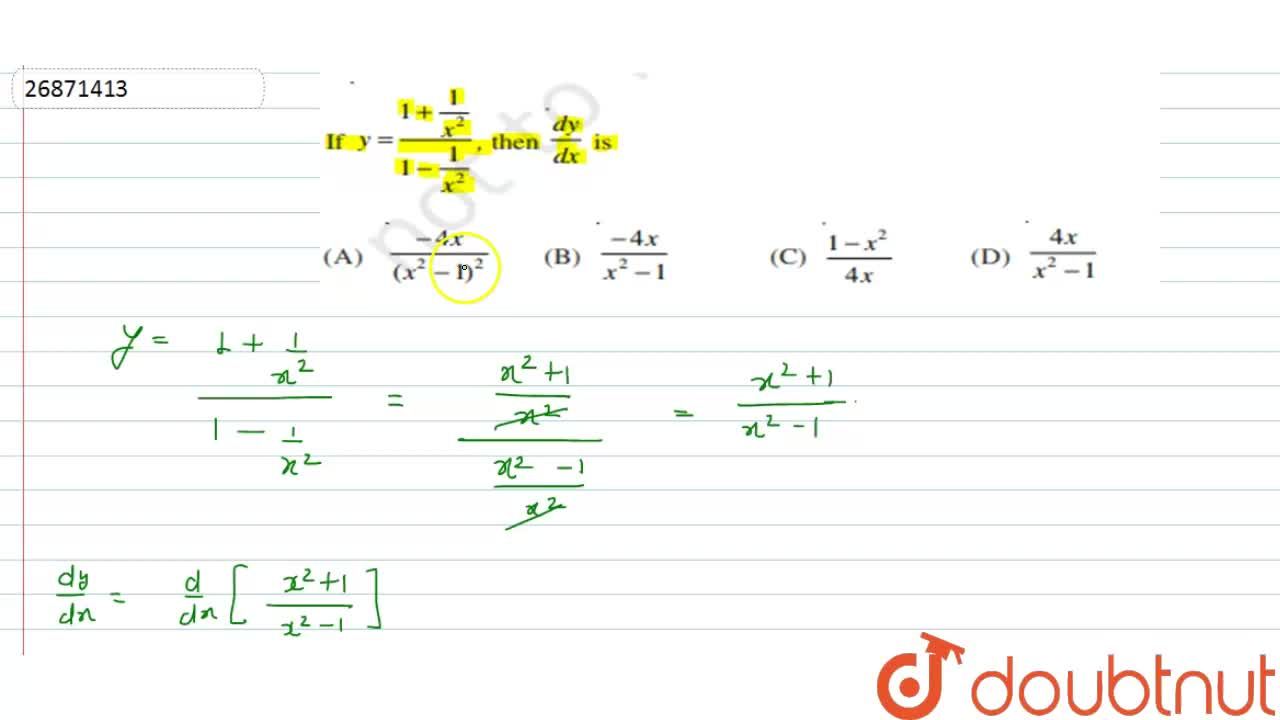
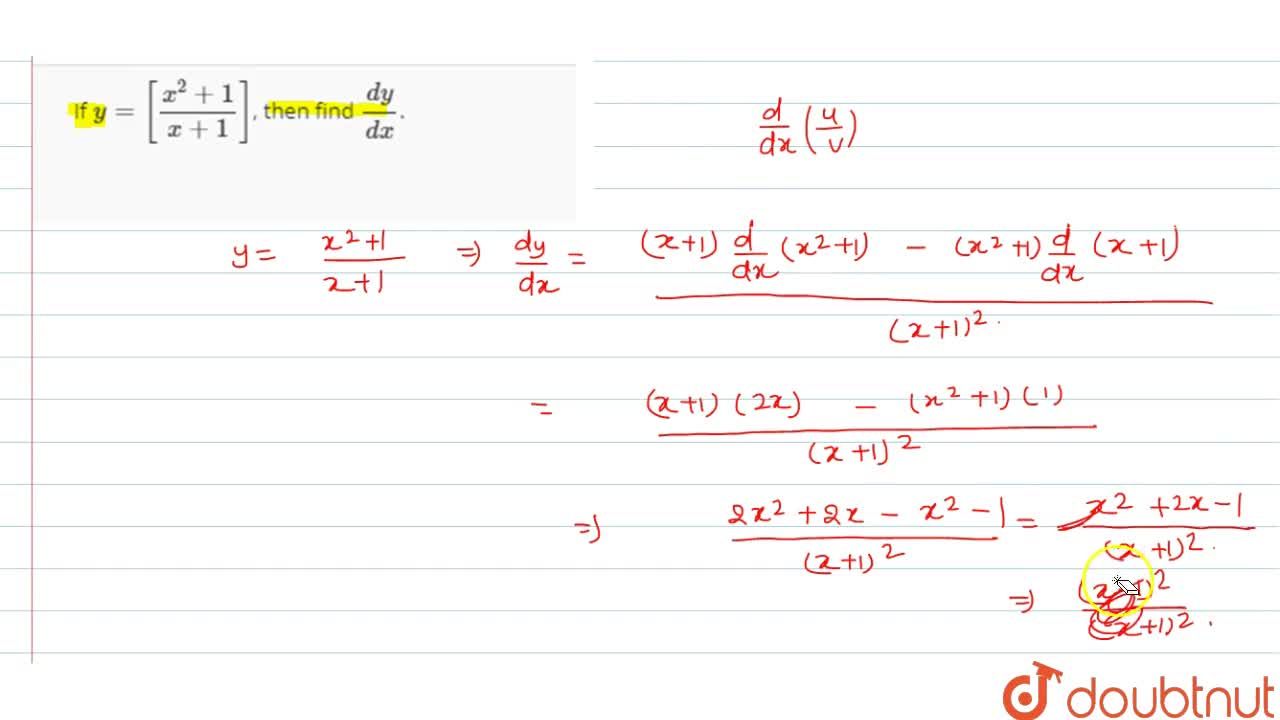
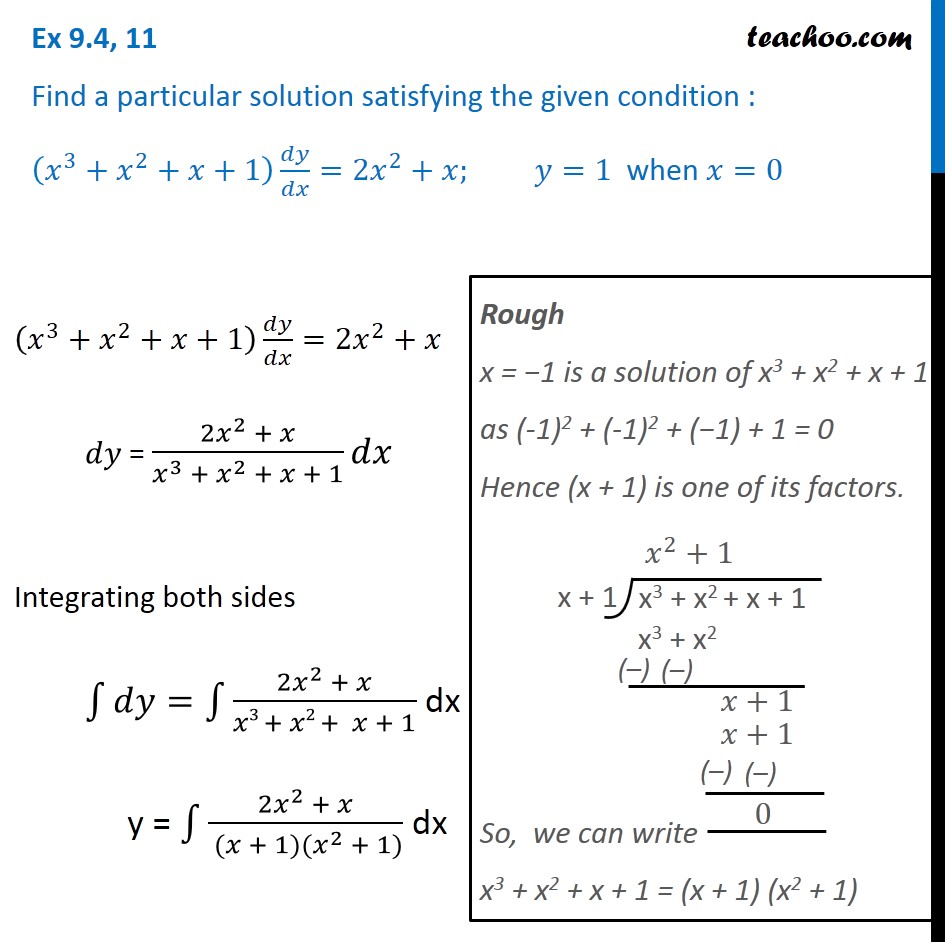
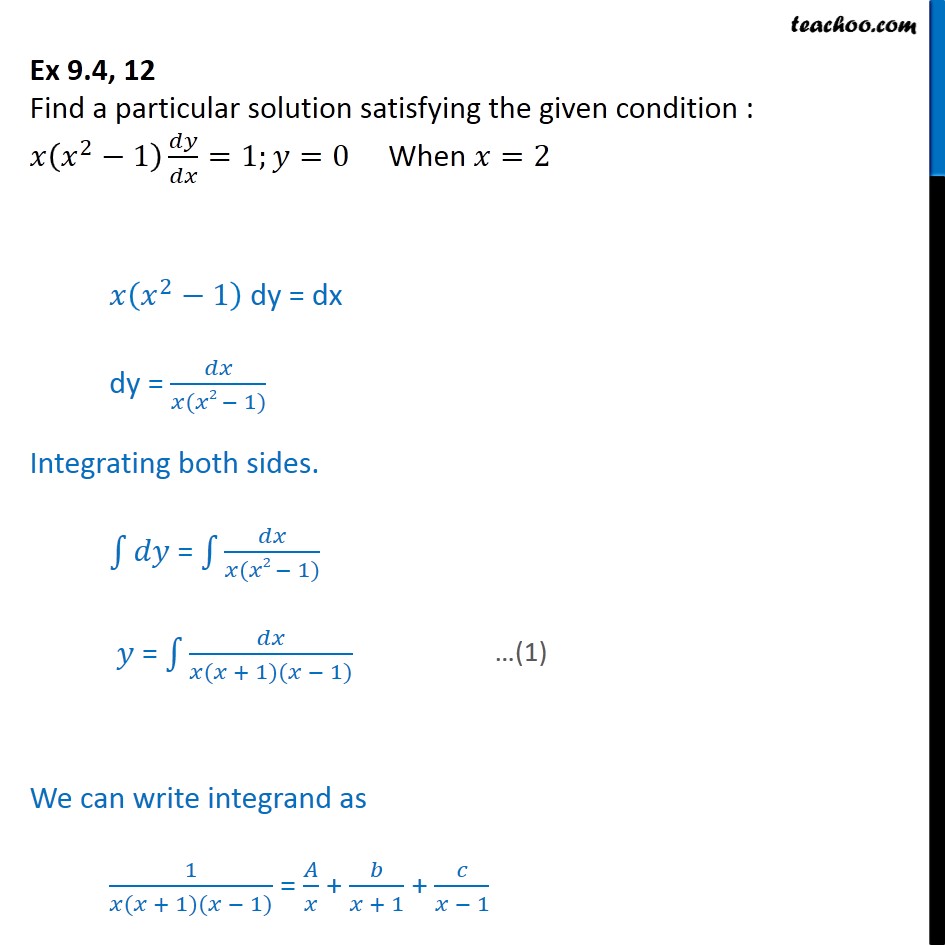
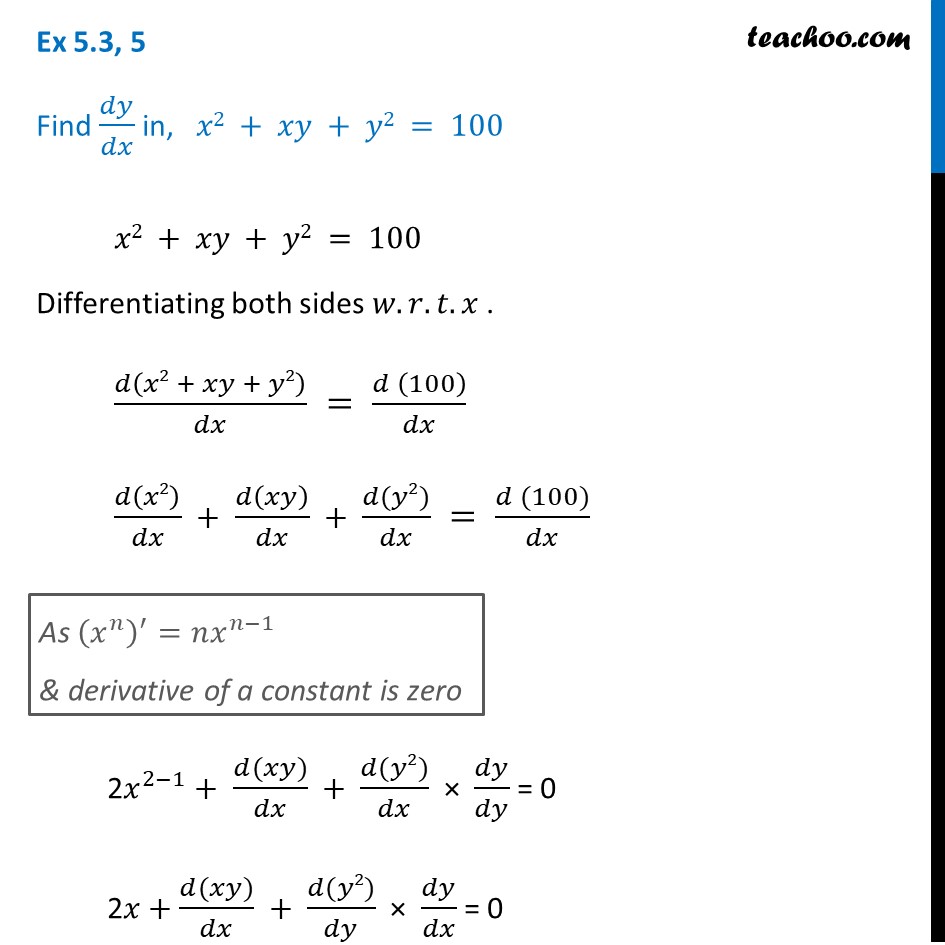
Find dy/dx if y=x^3-1/x+2-Use logarithmic differentiation to find dy/dx y= (x1) (x2) / (x1) (x2), x > 2 Ex 94, 12 Find a particular solution satisfying the given condition 𝑥 𝑥﷮2﷯−1﷯ 𝑑𝑦﷮𝑑𝑥﷯=1;𝑦=0 When 𝑥=2 𝑥 𝑥﷮2﷯−1﷯ dy = dx dy = 𝑑𝑥﷮𝑥(𝑥2 − 1)﷯ Integrating both sides ﷮﷮𝑑𝑦﷯ = ﷮﷮ 𝑑𝑥﷮𝑥(𝑥2 − 1)﷯﷯ 𝑦 = ﷮﷮ 𝑑𝑥﷮𝑥(𝑥 1)(𝑥 − 1)﷯﷯ We can write integrand as 1﷮𝑥(𝑥 1

Find dy/dx if y=x^3-1/x+2のギャラリー

各画像をクリックすると、ダウンロードまたは拡大表示できます

Ex 5 3 12 Find Dy Dx In Y Sin 1 1 X2 1 X2 Chapter 5
If Y 3 U 2 U And U 4x 1 X 2 Find Dy Dx Brainly In
If Y X 1 X Prove That 2x Dy Dx X 1 X Brainly In
If Y Root Of 1 X 1 X Then Prove That 1 X 2 Dy Dx Y 0 Brainly In
If 1 X 2 1 Y 2 A X Y Then Prove That Dy Dx
Ex 6 3 3 Find Slope Of Tangent Y X3 X 1 At X 2
If Y X X2 1 1 2 M Then Show That X2 1 D2y Dx2 X Dy Dx M2y 0 Mathematics Topperlearning Com 6jd9kell
Find Dy Dx Y X 2 1 3 2x 1 Sqrt X 3 4x 1
Example 25 Find Dy Dx If Y Sin Y Cos X Chapter 5 Class 12
Find Dy Dx For The Given Function Y X 1 X 2 X Brainly In
Chapter Differentiation Questions 1 Find The Value Of Answers 1 2 If F X 2 2x 5 Find F 2 Answers 2 3 If Y 2s And X 2s 1 Ppt Download
How Do You Find Dy Dx For Y 2x Sqrt X 1 Socratic
ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Find dy/dx if y=x^3-1/x+2」の画像ギャラリー、詳細は各画像をクリックしてください。

D Dx Formula
Find Dy Dx Y X X 1 X Brainly In
1
Ex 5 5 12 Find Dy Dx Xy Yx 1 Class 12 Cbse Ncert
Solved 2 Find Dy Dx If Y 3 Ind The First Derivative Of Chegg Com
1
If Y Log X 2 X 1 X 2 X 1 2 3 Tan 1 3x 1 X 2 Find Dy Dx Sarthaks Econnect Largest Online Education Community
Solved Find Dy If Y X X 2 4 Find Sigma I 1 30 I Chegg Com
If Y Sqrt X 1 Sqrt X Then Dy Dx At X 1 Is Equal To Youtube
Find Dy Dx When X 1 T 2 1 T 2 And Y 2t 1 T 2 Sarthaks Econnect Largest Online Education Community
Find Dy Dx If Y X 2 1 X 2 1 Brainly In
If Y 1 Root X X 1 2 Then Find Dy Dx Sahay Sir
ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Find dy/dx if y=x^3-1/x+2」の画像ギャラリー、詳細は各画像をクリックしてください。

If Log 1 X 2 X Y 1 X
Find Dy Dx For Y Sin 1 2xsqrt 1 X 2 1 Sqrt2 X 1 Sqrt2 Mathematics Shaalaa Com
If Y 1 1 X 2 1 1 X 2 Then Dy Dx Is Equal To
Find Dydx If Y Sec 1 12x 2 1 0 1 2
Ex 5 3 14 Find Dy Dx In Y Sin 1 2x Root 1 X2 Cbse
If Y Log E Tan 1 1 X 2 Find Dy Dx
1 If Y Sqrt X 2 1 Log 1 X Sqrt 1 1 X 2 Find Dy Dx 2 Find The Equation Of Tangent To The Curve Y Sqrt 4x 2 Which
Ex 6 3 2 Find Slope Of Tangent Y X 1 X 2 At X 10
Ex 5 7 17 If Y Tan 1 X 2 Show X2 1 Y2 2x X2 1
Solved Find Dy Dx A Y X 2 B Y X Y 2 C Y X X Chegg Com
Find Dy Dx If Y Sin 1 2x 1 X 2
Solved D Dx 1 X 3 1 X X 2 At X 1 Is 0 4 6 6 2 Chegg Com
ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Find dy/dx if y=x^3-1/x+2」の画像ギャラリー、詳細は各画像をクリックしてください。

If Y E X Sin 2 X Sin X X Find Dy Dx With Video Teachoo
Misc 13 Find Dy Dx If Y Sin 1 X Sin 1 Root 1 X2 Miscellaneous
Example 47 Find Dy Dx Where Y At 1 T X T 1 T 2
If Y 1 X 1 X 2 1 X 4 1 X 2n Then The Value Of Dydx At X 0 Is
If Y 1 X 2 3 Then Dy Dx
If Y X 2 1 Log 1 X 1 1 X 2 Find Dy Dx Sarthaks Econnect Largest Online Education Community
Solved Section 3 1 6 9 16 25 Section 3 2 7 11 15 Chegg Com
If Y X X 2 Prove That X Dy Dx 1 Y Y Sarthaks Econnect Largest Online Education Community
Ex 5 3 13 Find Dy Dx In Y Cos 1 2x 1 X2 Ncert Ex 5 3
Iit 1980 Find Dy Dx If Y 5 3 Sqrt Square 1 X Squarecos 2x 1 Youtube
If Y Tan 1 2x 1 X 2 Then Find Dy Dx
If Y Log X 1 X2 1 2 Prove That Dy Dx 1 Log X 1 X2 1 2 1 1 X2 1 2 Mathematics Topperlearning Com Qsw6n0xx
ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

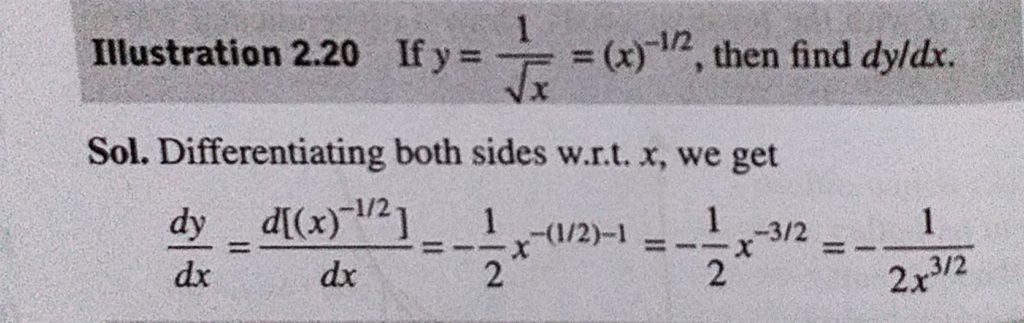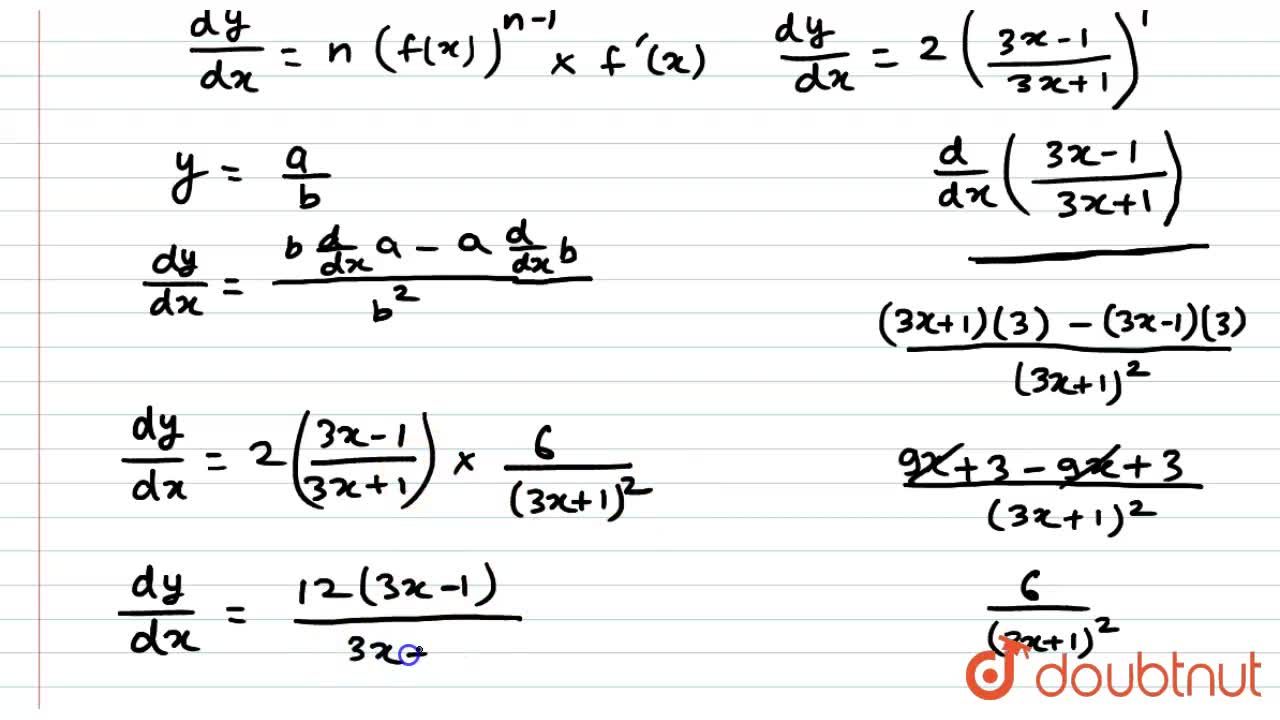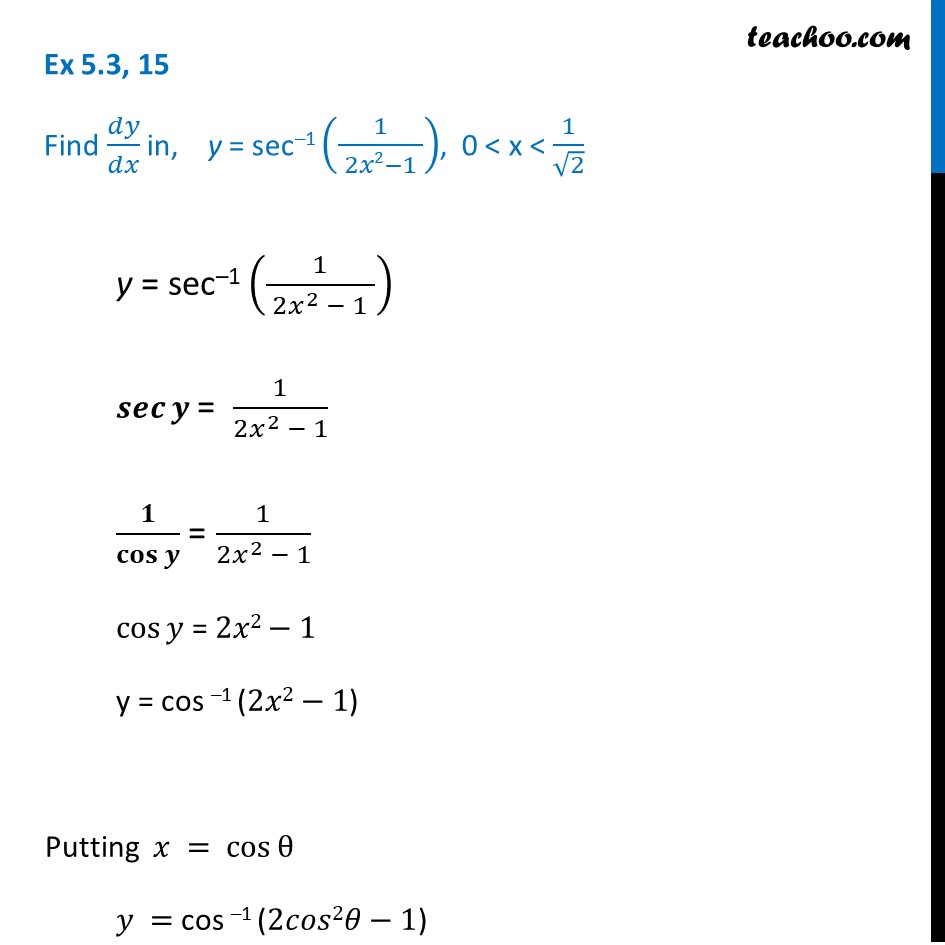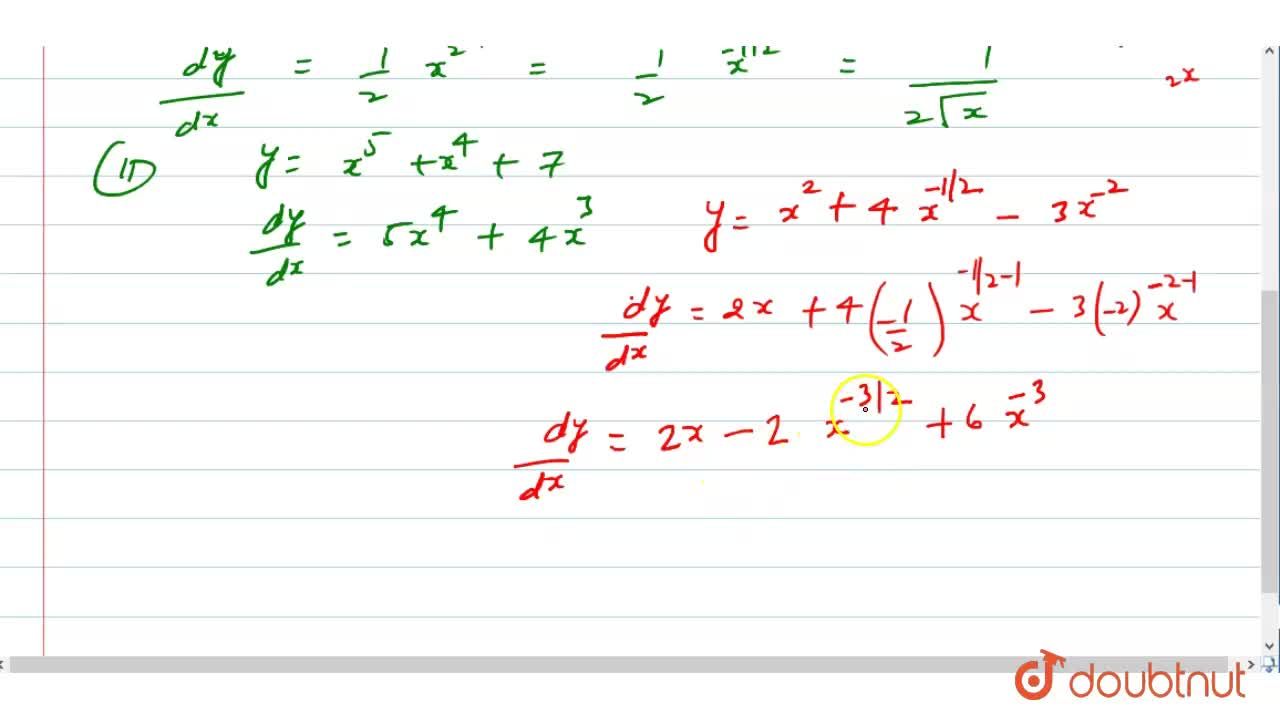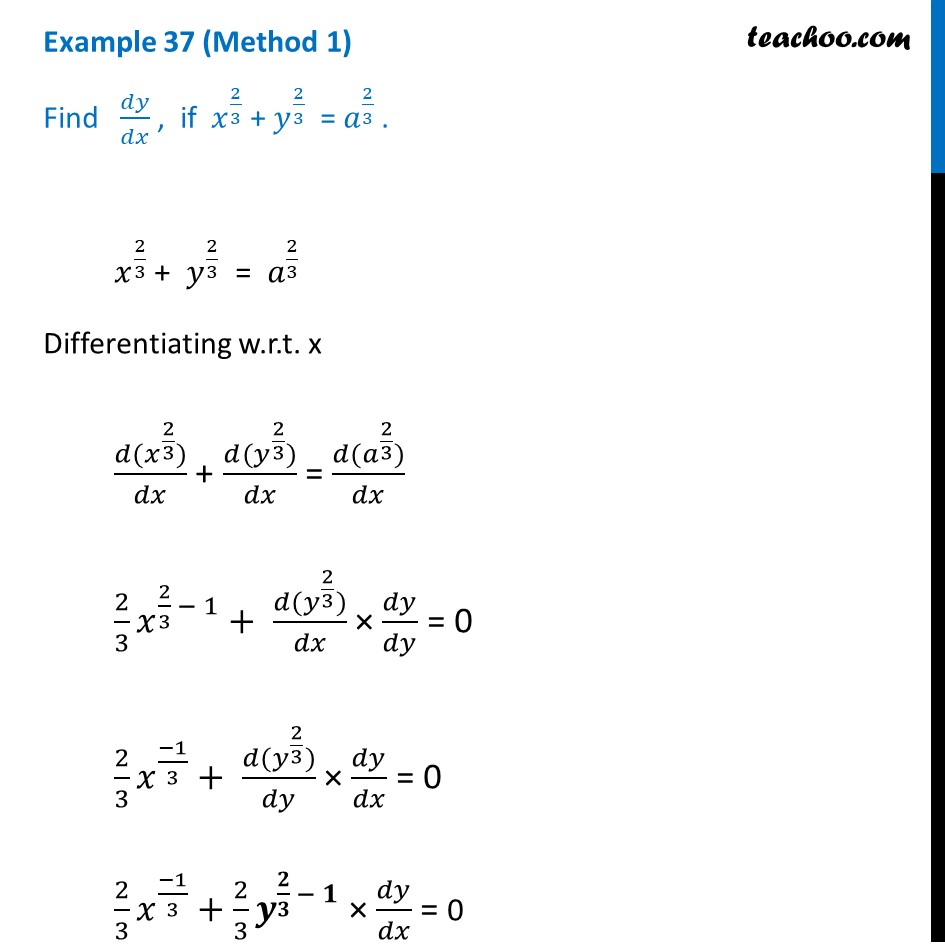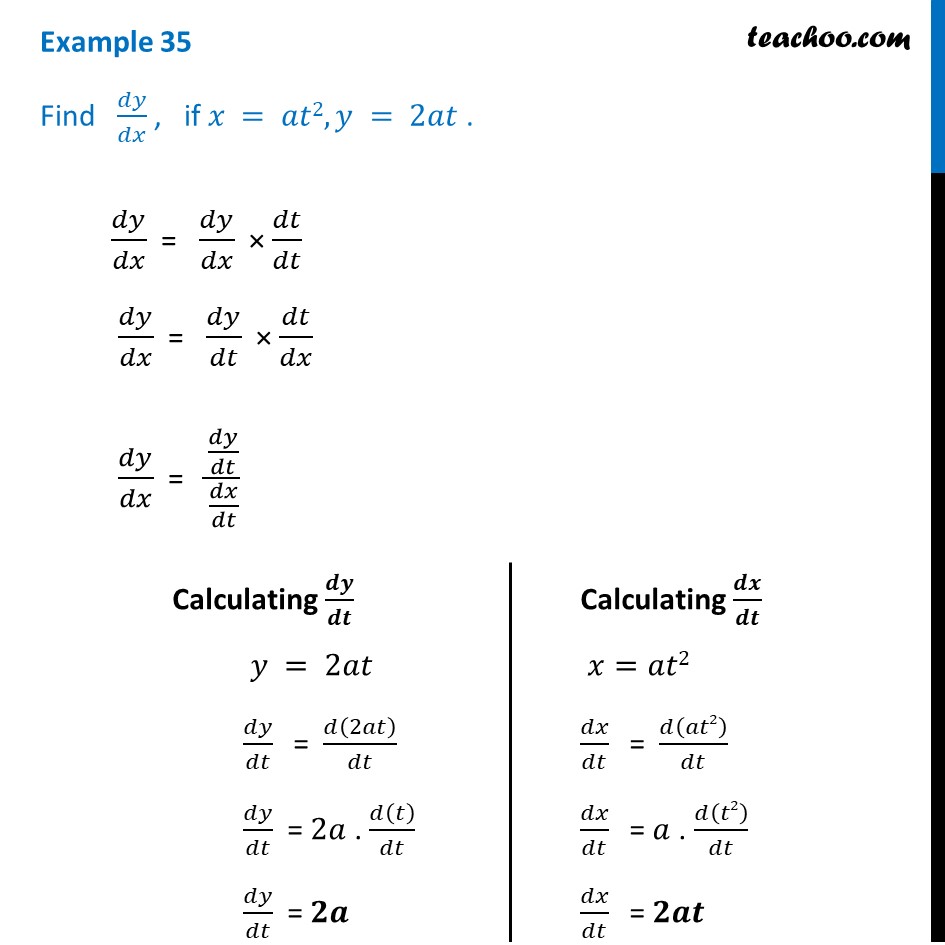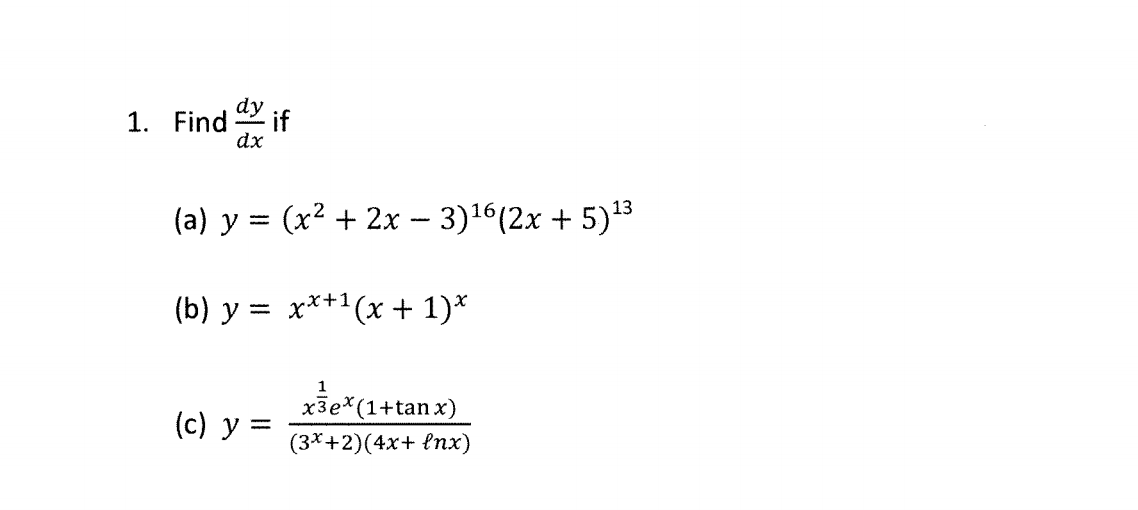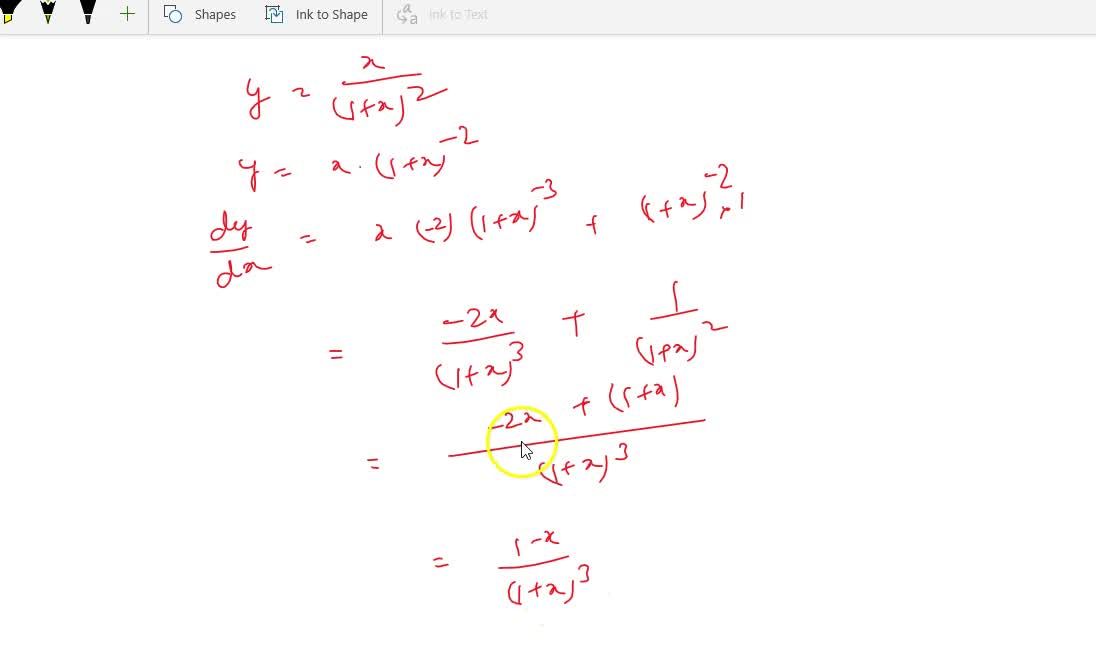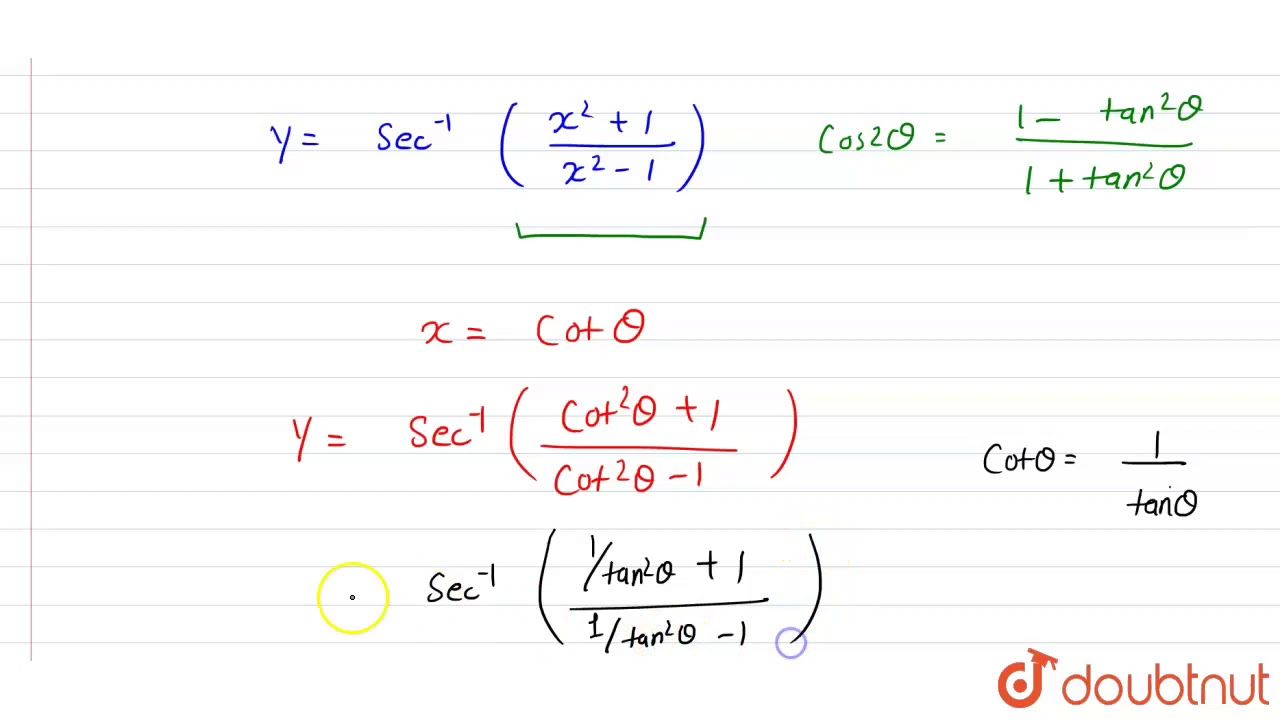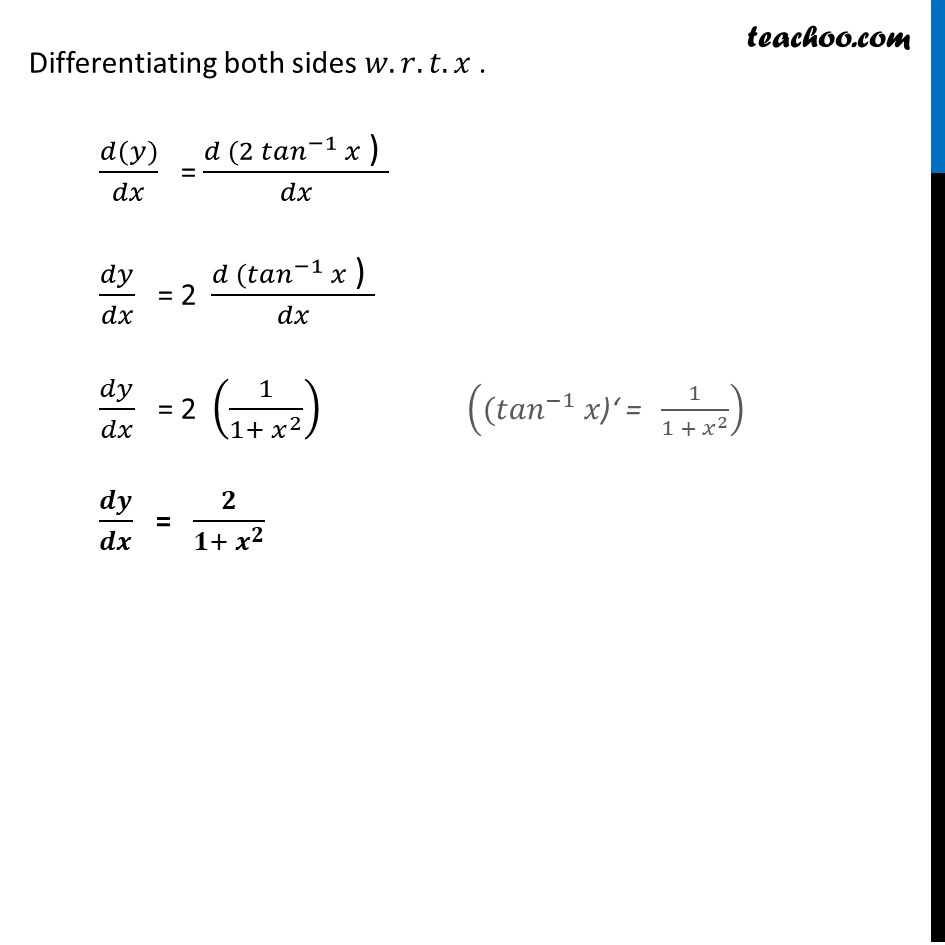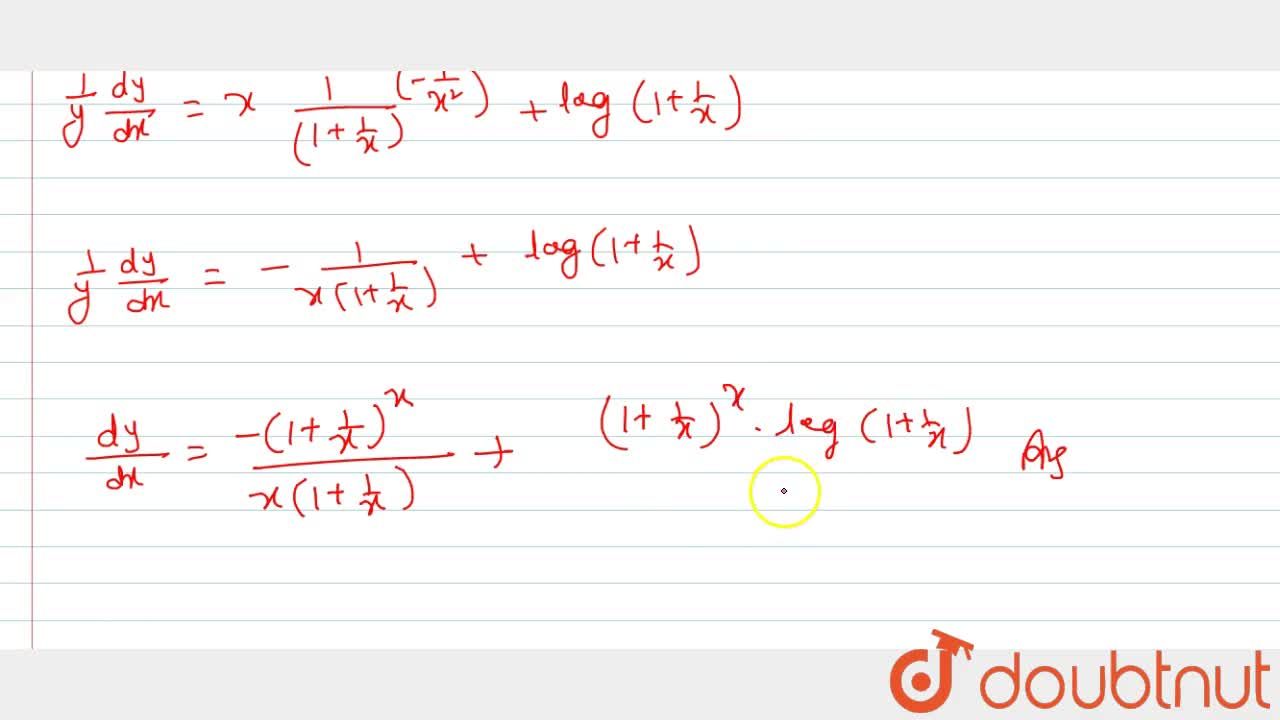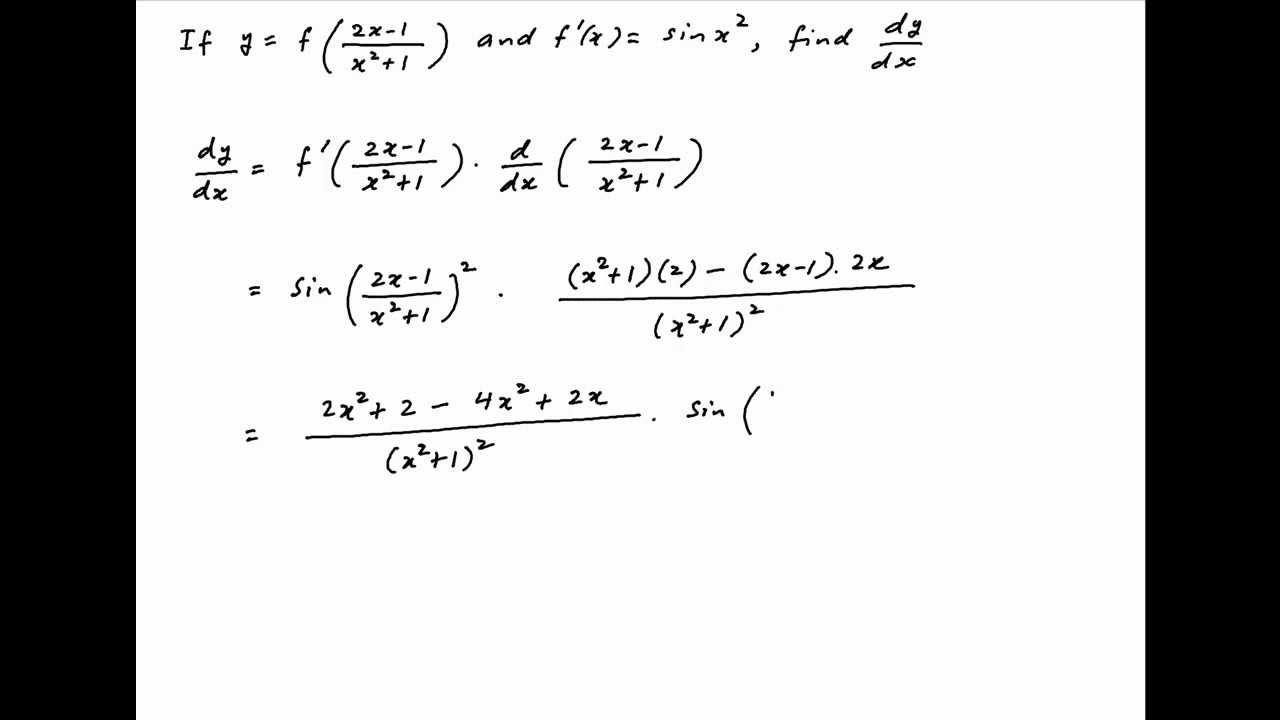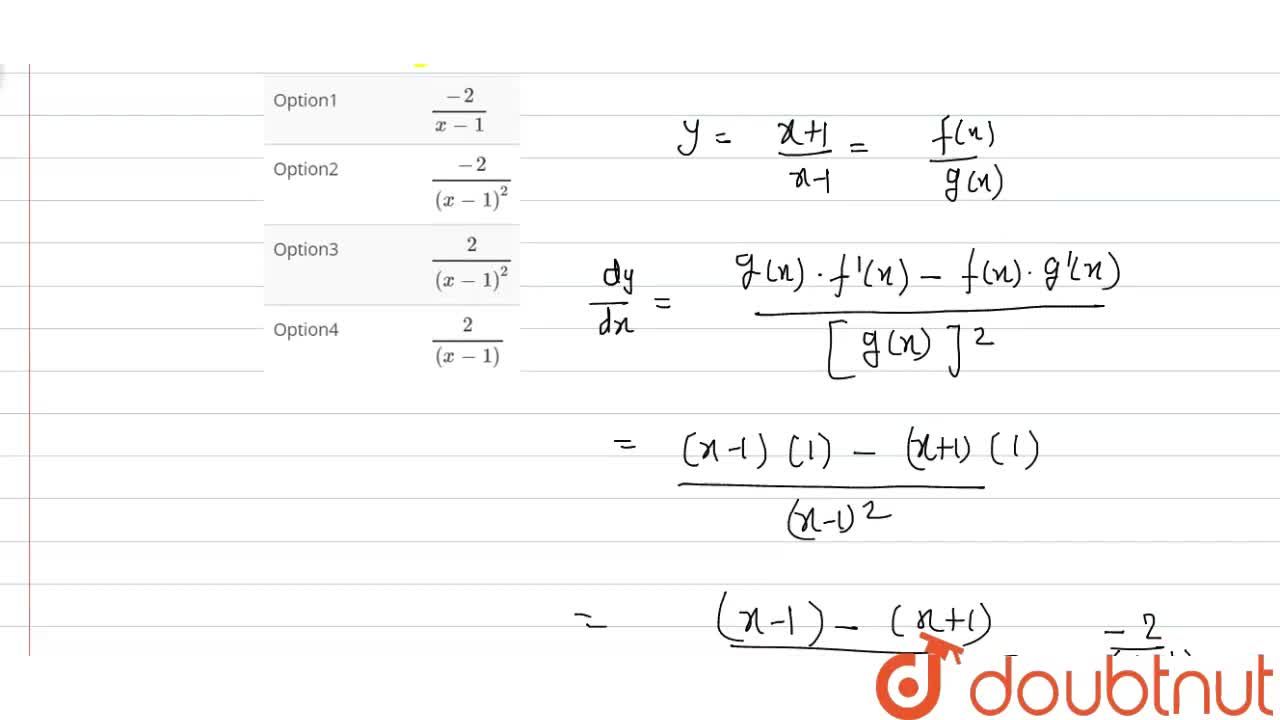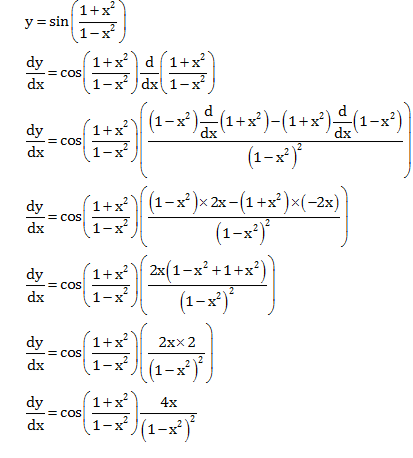「Find dy/dx if y=x^3-1/x+2」の画像ギャラリー、詳細は各画像をクリックしてください。

Solved Find Dy Dx Given That Y Ln Squareroot X 2 1 Ln X Chegg Com
How To Find Dy Dx Of The Function Y X 1 X 2 X 1 2 Quora
Ex 5 7 12 If Y Cos 1 X Find D 2y Dx 2 In Terms Of Y Alone
How To Find Dy Dx Of The Function Y X 1 X 2 X 1 2 Quora
Use Chain Rule To Find Dy Dx If Y 3x 1 3x 1 2
If Y X 2 2x 3x 4 Then Find Dy Dx Youtube
Solved Assignment 1 If Y Xsinx Find Dx Dy 2 If Y Chegg Com
Ex 5 3 15 Find Dy Dx In Y Sec 1 1 2x2 1 Chapter 5
Ex 5 3 5 Find Dy Dx In X2 Xy Y2 100 Class 12
If X 1 Y Y 1 X 0 Then Prove That 1 X 2 Dydx 1 0
Find Dy Dx When I Y Sqrt X Ii Y X 5 X 4 7 Iii Y X 2 4x 1 2 3x 2
If Y X X 1 X 2 Then Dy Dx Is Brainly In
ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Find dy/dx if y=x^3-1/x+2」の画像ギャラリー、詳細は各画像をクリックしてください。

If Y Log X X 2 A 2 X 2 A 2 X Find Dy Dx Target Batch
Example 37 Find Dy Dx If X2 3 Y2 3 3 Ncert Examples
Example 35 Find Dy Dx If X At2 Y 2at Class 12 Cbse
Solved Find Dy Dx If Y X 2 2x 3 16 2x 5 13 Y Chegg Com
Find Dy Dx If Y X 1 X 2
If Y 1 X Find Dy Dx Also Find The Value Of X For Which Dy Dx Becomes Undefined
Ex 5 3 15 Find Dy Dx In Y Sec 1 1 2x2 1 Chapter 5
Solved Integral 1 4 Ln X X 2 Dx If Dy Dx 2y 2 And If Y Chegg Com
If Y Sec 1 X 2 1 X 2 1 Then Dy Dx Youtube
Ex 5 3 11 Find Dy Dx In Y Cos 1 1 X2 1 X2 Ex 5 3
Find Dy Dx Of Y 1 1 X X
Solved Untuuvily 11 Laupuises Or 99 And 1 9 18 Find Dy Dx Chegg Com
ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Find dy/dx if y=x^3-1/x+2」の画像ギャラリー、詳細は各画像をクリックしてください。

1
Iit 19 Find Dy Dx If Y F 2x 1 Square X 1 And F X Sin Square X Youtube
If Y X 1 X 1 Then What Is Dy Dx Equal To
Find Dy Dx Y Sin 1 X2 1 X2 Mathematics Topperlearning Com 8s1l799
If Y X 2 1 X 1 Then Find Dy Dx Sahay Sir
If Y X 1 X Show That 2x Dydx Y 2 X
Ex 5 5 12 Find Dy Dx Xy Yx 1 Class 12 Cbse Ncert
Solved Find Dy Dx If Xy 2 Xy 2 E Y 3x X 3 Find The Chegg Com
Example 41 If Y Sin 1 X Show That 1 X2 D2y Dx2 X Dy Dx 0
Solved Find Dy Dx For Y X 3 Squareroot X 1 3x 2 2 Chegg Com
If Y X 2 1 X 1 Then Find Dy Dx
Find Dy Dx In The Following Y Sin 1 1 X 2 1 X 2 0 Lt X Lt 1
ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Find dy/dx if y=x^3-1/x+2」の画像ギャラリー、詳細は各画像をクリックしてください。

Example 33 Find Dy Dx If Y X X Y X X A B Teachoo
Ex 5 5 16 Find Derivative Of F X 1 X 1 X2 1 X4 1 X8
Solved Use Logarithmic Differentiation To Find Dy Dx If Y Chegg Com
Solved 2 Find Dy Dx If Y 3 Ind The First Derivative Of Chegg Com
Ex 9 4 11 Find Particular Solution X3 X2 X 1 Dy Dx
If Y Cos 1 1 X 1 X Then Find Dy Dx Youtube
Find Dy Dx If Y Tan Inverse Square Root Of 1 X 2 Square Root Of 1 X 2 Square Root Of Maths Continuity And Differentiability Meritnation Com
Ex 5 3 9 Find Dy Dx In Y Sin 1 2x 1 2x2 Chapter 5
If Y 1 A 2 X 2 Find Dy Dx Brainly In
If Y Sqrt 1 X 1 X Then Dy Dx Equals Youtube
Ex 9 4 12 Find Particular Solution X X2 1 Dy Dx 1 Y 0
Ex 5 3 5 Find Dy Dx In X2 Xy Y2 100 Class 12
ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「Find dy/dx if y=x^3-1/x+2」の画像ギャラリー、詳細は各画像をクリックしてください。

Find Dy Dx If Y X 2 1 X 2 1
If Y Sin X 2 Cos X 2 2 Find Dy Dx At X Pi 6 Youtube
Solved 1 For 1 12 Find Dy Dx For 1 Y Xe 3x 2 Y Chegg Com
Find Dy Dx At X 1 Y Pi 4 If Sin 2 Y Cos Xy K Sarthaks Econnect Largest Online Education Community
If Ylog X X Y Prove That Dydx Logx 1 Logx 2
Misc 23 If Y Ea Cos 1 X Show 1 X2 D2y Dx2 X Dy Dx
If Y Sinx X Sin 1 X 1 2 Find Dy Dx Explain In Great Detail Do Not Go Shortcut Mathematics Topperlearning Com W0t3auii
If Y E Acos 1x 1 X 1 Show That

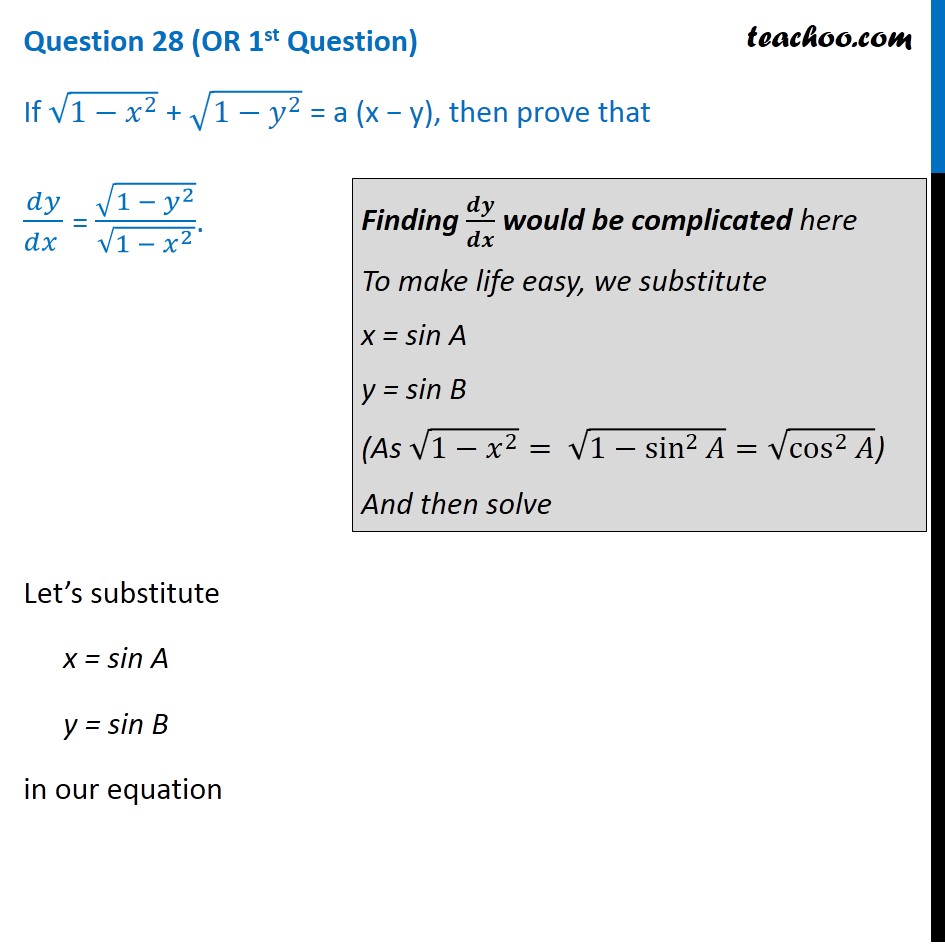
 Solve dy/dx = (y^2 1)/ (x^2 1), y (2) = 2 Relevant Equations dy/dx = (y^2 1)/ (x^2 1), y (2) = 2 This is my attempt Since y (2) = 2, So, Is this ok, or did I make any mistake?Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!

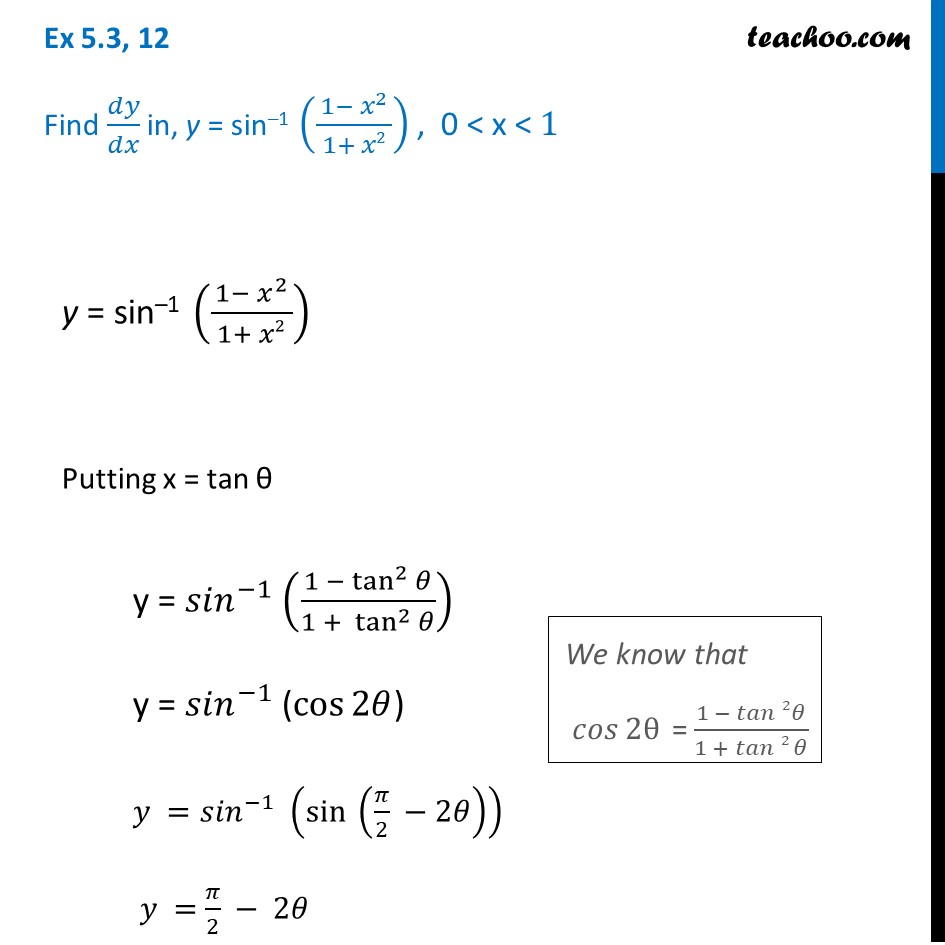
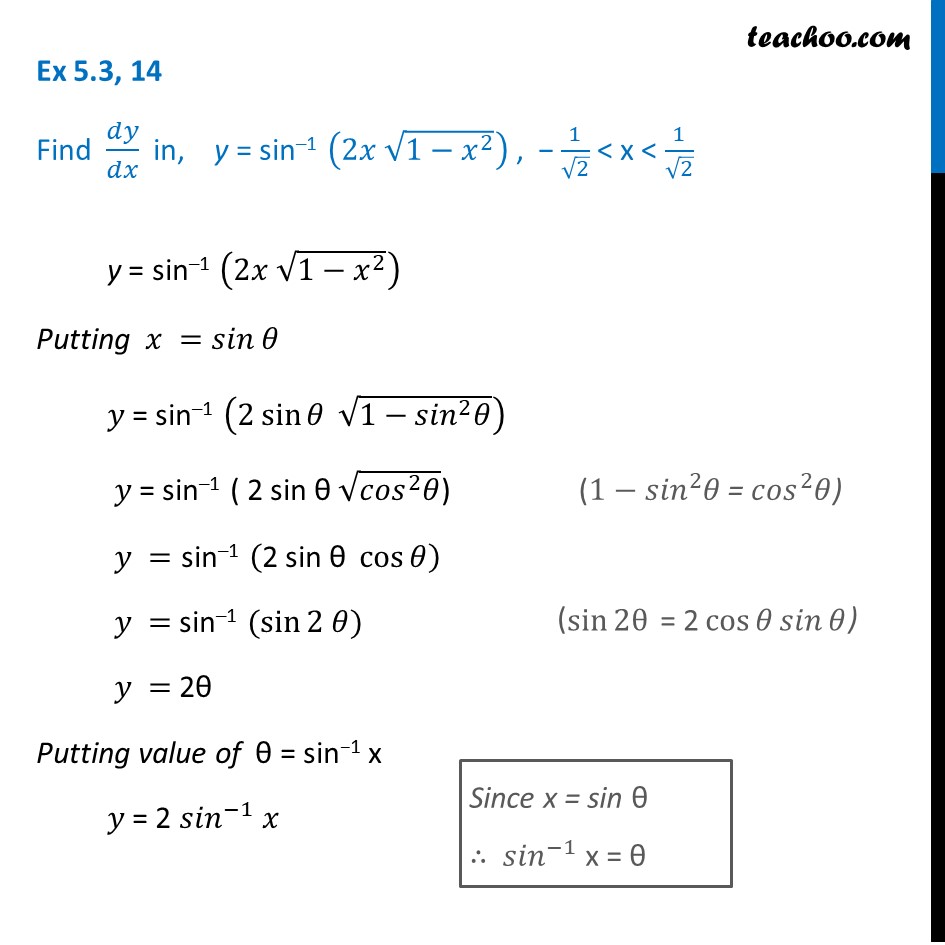
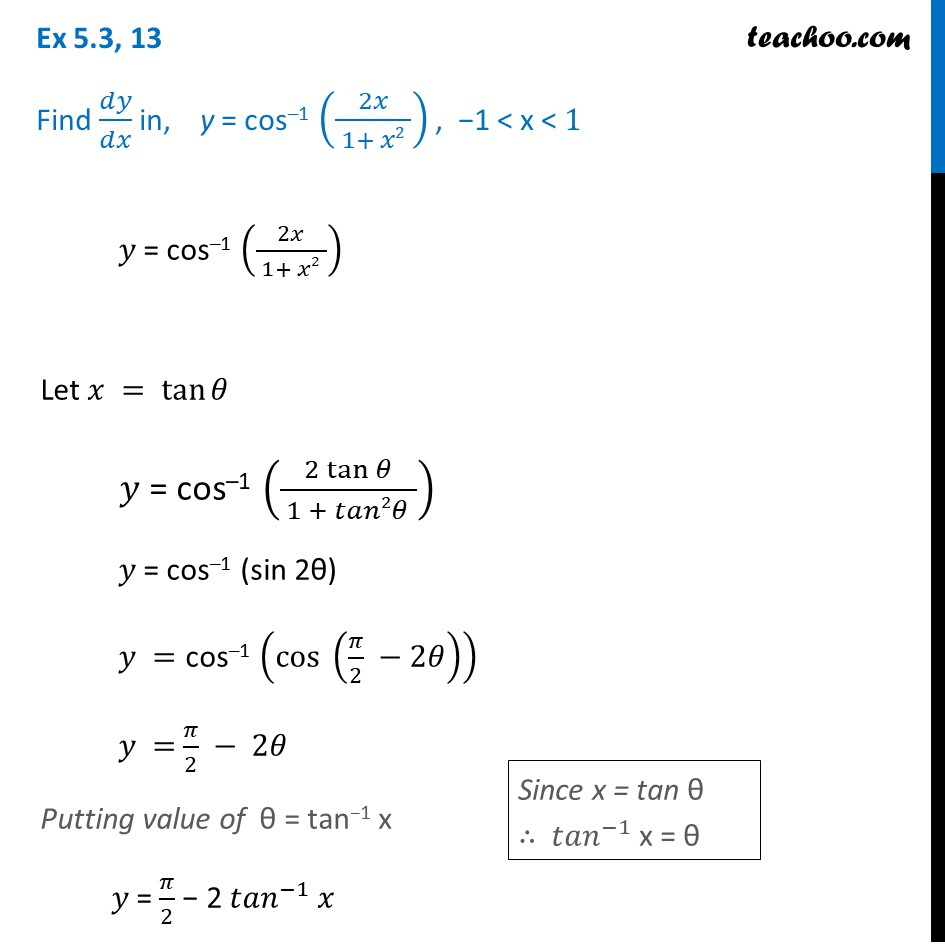
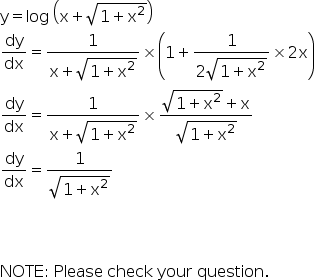
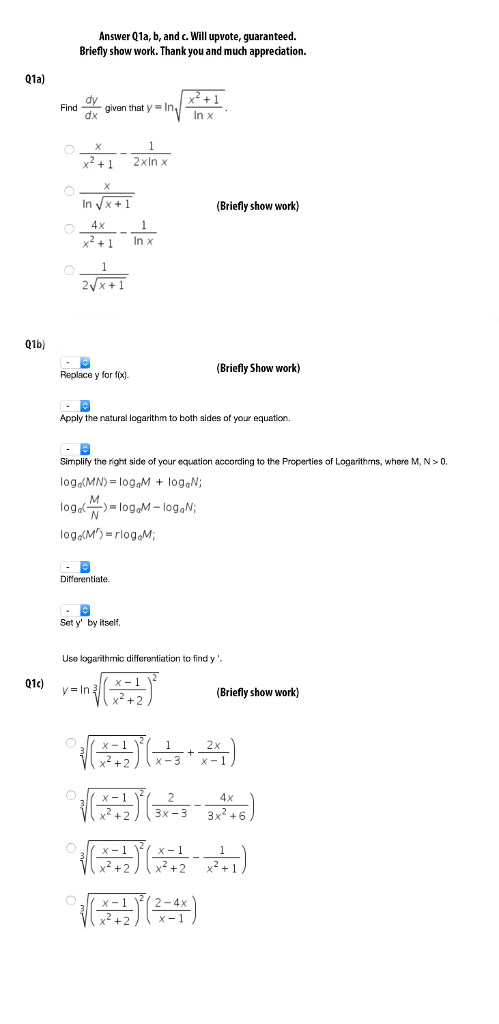
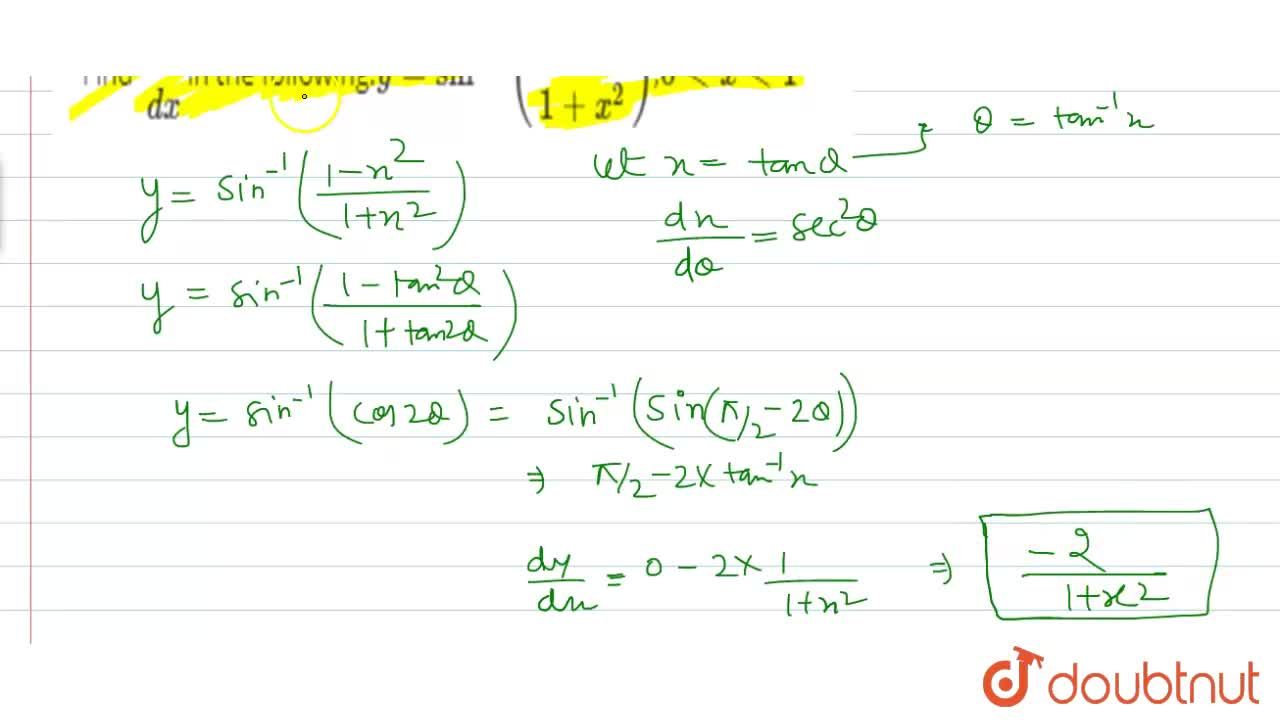
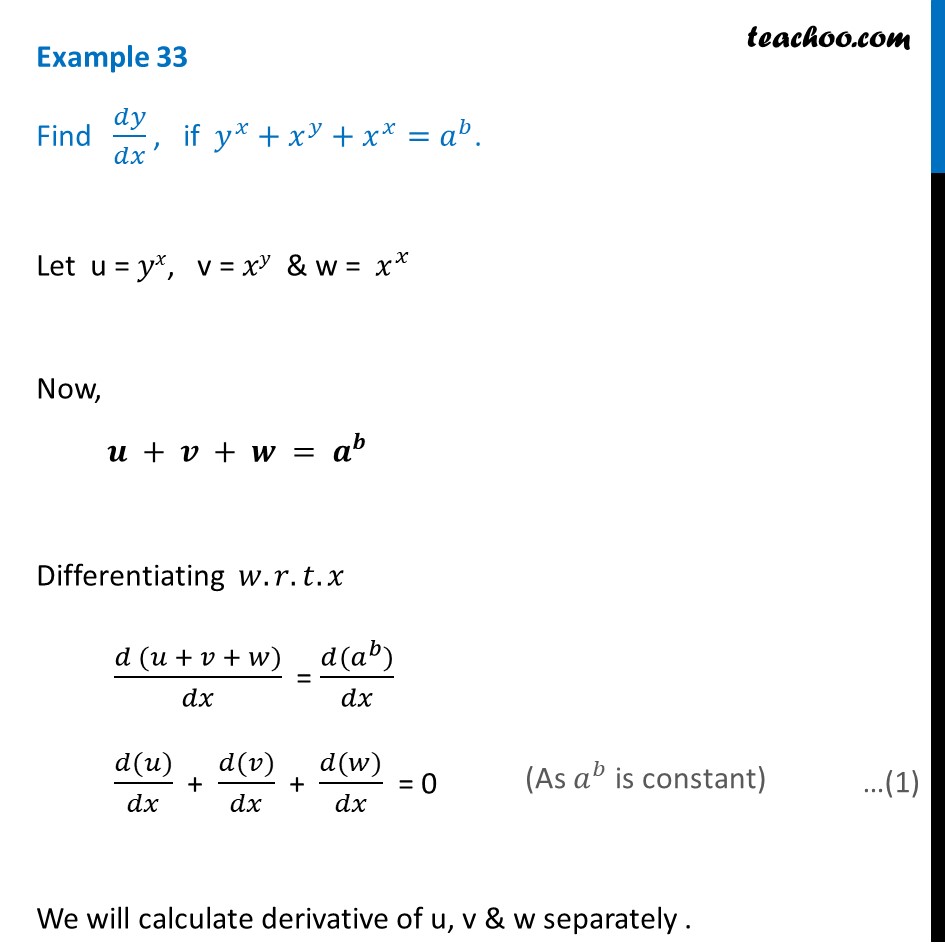
Incoming Term: find dy/dx if y=(x+1)(x+2), find dy/dx if y=(x-1)(x-2)/√x, find dy/dx if y=x^3-1/x+2, find dy/dx if y=log(1-x^2/1+x^2), find dy/dx if y=cot^-1(x^2), find dy/dx when y = x^2sinx/1-x, find dy/dx if y= x3-1/x+2, find (dy)/(dx) if y=cos^(-1)((1-x)/(2 sqrt(x))), find dy/dx if y=sin^-1(1-x^2/1+x^2), find (dy)/(dx) if y=e^(tan^(-1)x^(2)),

コメント

コメントを投稿

人気の投稿

[最新] 田中はゆま ブログ 288337-田中はゆま ブログ

イメージ
田中理恵 公式ブログ Home 本日、2月22日(月)の2100〜2154まで放送の、日本テレビ「人生が変わる1分間の深イイ話 」に出演します！田中光敏拝 管理人です、動画を集めてみました。 ①受賞後すぐの控室での動画です。 公式サイト、Facebookページのみなさんにも すぐにご報告をと思って監督が録画したものです。 ②キネマ旬報ベスト・テン発表＆表彰式全体②スレッドをたくさん立てる これはJMeterの実行でリソース使いすぎるのではという話もあるがどうなのか？ JConsoleというので、JMeterのスレッド数を見てたけど使われたスレッドは破棄されていったように見えるが。 jmxの構成は以下のような感じ。 スレッドグループA ※スレッド数100 新保海鈴のjリーグ初ゴールはママ マリアに捧げる 父と親子対決なるか Mediaログ 田中はゆま ブログ
続きを読む

画像 沖縄 県 サッカー キャンプ 286294-沖縄 県 サッカー キャンプ 2021

イメージ
沖縄サッカーキャンプ 「いいね！」3件 沖縄県が誘致する沖縄サッカーキャンプのページです。沖縄サッカーキャンプ 3 likes 沖縄県が誘致する沖縄サッカーキャンプのページです。「☆accs（アックス）サッカークラブ☆ 幼児～小学生沖縄県サッカー専門スクール＆サッカークラブ」のブログトップページです。☆中部地区・北部地区☆幼児（3歳）〜 小学生（12歳）専門サッカー教室☆ ※名護地区は新たに選手登録コースを設立！ 沖縄メディア サッカーニュース 1月 ジュニアサッカーnews 沖縄 県 サッカー キャンプ 2021
続きを読む

[新しいコレクション] 大空 鳥 314712-大空 鳥

イメージ
已售出 30 (大鳥叔叔模型)田宮TAMIYA 3支套組模型專用基礎筆平筆NO01 平筆NO3 面相筆 小 $95 已售出 944 (大鳥叔叔模型) TAMIYA 田宮871速乾型超薄膠水 模型細部專用 $110 已售出 293お問合わせの際はぐるなびを見たというとスムーズです。 ネット予約はこちらから 住所 〒 兵庫県神戸市長田区若松町4215社會 社會焦點 保障人權 台中男「露鳥狂搓」下秒液體噴射亂甩！ 她見衣服一片白濁崩潰 阿傑被依法判處拘役50日。 （圖／示意圖，無關本案 大空直美 萌娘百科万物皆可萌的百科全书 大空 鳥
続きを読む